LEARNING OBJECTIVE 3.A.1.1 - Express the motion of an object using narrative, mathematical, and graphical representations. AP MECHANICS -C LEARNING OBJECTIVE INT 2.A -Calculate relationships among the radius of a circle, the speed of an object (or period of revolution), and the magnitude of centripetal acceleration for an object moving in uniform circular motion.
When an object performs a uniform circular motion, the motion can then be described not only by its constant speed v but also by specifying how much time T it takes to complete a full circle and also if it is making a faster revolution how many total full rotation it is making per second. This kind of new way of describing the motion is easier as these variables are easy to observe in practice. For example, we can describe the motion of Moon rotating in a circular motion around Earth by specifying the number of days it takes for the Moon to rotate a full circle around Earth and it in a sense acts as a proxy to Moon’s speed. Let’s look at two such useful ways of describing circular motion and how they can be related to variables such as speed v we have used so far.
Period of revolution
When an object is performing a uniform circular motion, we can simply ask how much time in seconds it is taking the object to complete one full revolution and call it a period of revolution or simply Period denoted by symbol T. For example, if it is taking an object 10 seconds to complete a full rotation its period is T = 10 seconds. We can also choose to denote the period in other units for example it takes about 27 days for the Moon to complete and full rotation around Earth so its period is T = 27 days (approximately). Neatly we can say, Period : Time required for one revolution.
Frequency
When an object is performing a circular motion we may be also interested in knowing how many total revolutions it is performing in a unit of time. If we choose the unit of time to be seconds then we count how many revolutions it performed during a second. If we choose a unit of time to be minutes we simply count how many revolutions it performed in a minute and so forth. it is just another way of describing the motion and we call it Frequency: Number of revolutions per unit time, denoted by symbol f. Obviously, the Period and frequency are two descriptions of the same motion and there is a simple relationship between them. Suppose an object takes 0.1 seconds to complete one full revolution then the period T = 0.1 seconds, and then if we ask how many revolutions it will do in one second and answer will be 1/0.1 = 10 and that will be the frequency f. Hence we can deduce:
$f = \frac{1}{T}$
Or frequency is simply inverse of T and can be measured in units $T^{-1}$, We have a special name for such a unit and it is called Hertz or in short Hz.
Finding relationships between variables
We have so far defined several variables that can describe the motion of an object moving in a circle with uniform speed. The table below outlines them.
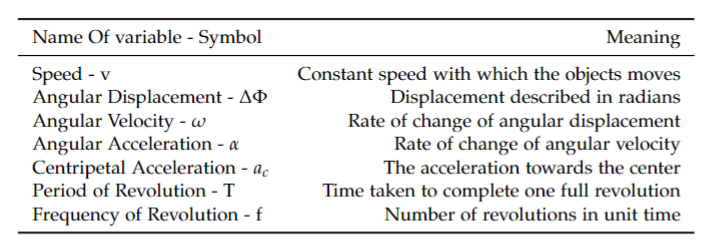
Let’s now find some equations relating these variables to each other. This will allow us to shift from one variable to another if required in a problem.
We have already defined the relationship between T and f in the previous section. Here are several other relationships that could be helpful.
Relation between V and $\omega$
If we look at the definition of speed v we find that it is simply how much the body travels along the circle in unit time. So we can say if the body travels the complete circle or full revolution in time t then the v = 2$\pi r$ / t. Now we express the same motion in terms of angular quantity $\Delta\Phi$ that will represent the motion of one complete revolution if $\Delta\Phi$ = $2\pi$ , i.e. the angle covered in one full circle, hence the angular velocity is $\omega$ = $2\pi$/t. Allowing us to deduce the relationship between v and $\omega$
$v = r \omega$
Relation between $a_c$ and $\omega$
If we start with the value of centripetal acceleration $a_c$ = $v^2 / r$, if we substitute our newfound value of v in terms of $\omega$ into this, we will get
$a_c = \omega^2 r$
Relation between V and T
v is simply the constant speed through which the object moves around the circle, T is the time it takes to complete one revolution and the distance travelled by an object in one revolution is $2\pi r$ hence using the definition of speed we can say,
$v = \frac{2\pi r}{T}$
Relation between V and f
Frequency f is related to T with a relationship f = 1/T or T = 1/f, if we substitute the same in the last equation we will get,
$v = 2\pi r f$
In the next section we will look into dynamics of circular motion.
