Mechanical energy and its conservation

LEARNING OBJECTIVE 3.4.B - Describe the behavior of a system using conservation of mechanical energy principles.
AP MECHANICS -C LEARNING OBJECTIVE CON 2.A -. Describe physical situations in which mechanical energy of an object in a system is converted to other forms of energy in the system.
Describe physical situations in which the total mechanical energy of an object in a system changes or remains constant.

Based on arguments developed in the last sections we can say that when the work is done either converted into kinetic energy or potential energy or both.

Kinetic energy and potential energy put together are called Mechanical energy.

Mechanical Energy = Kinetic Energy
+ Potential Energy

Mechanical Energy

When we throw an object up in the air, at the time when it just leaves our hand all the work done by us is converted into kinetic energy of the object. As the object continues to move upwards some of this kinetic energy starts to convert into potential energy and more specifically gravitational potential energy. At the top of the trajectory, the kinetic energy becomes zero and all of it gets converted to the potential energy. ‘

When the object starts its journey downwards this potential energy starts to convert back into kinetic energy and when the ball comes back to its position of projection potential energy becomes zero again and all of it is converted to kinetic energy, exactly the same amount the object started with. This means the sum of kinetic energy and potential energy conversion cannot exceed the total work done.

The law of kinetic energy states that work we do on an object eventually changes its kinetic energy:

$W = \Delta KE$

We just discovered that it is also possible that some of it also gets converted to potential energy. Let’s represent potential energy with U and its change as $\Delta U$.

In order to incorporate our new understanding we will need to modify the above equation to a new one :

$W = \Delta KE + \Delta U$

This means once an external force does work on a system it causes a change in kinetic and potential energies such that their sum is always equal to the work done by external force. In other words, if there are no more external forces acting on the system the total mechanical energy will not change or remain constant.

Hence, in a closed system, where no external forces are acting or doing work, the mechanical energy remains constant or conserved.

$ KE + U = \mbox{ Constant } $

The equation $ KE + U = \mbox{ Constant } $ represents one of the important laws in physics called the law of conservation of mechanical energy.

Law of conservation of mechanical energy

The energy can be converted from one type to another or can be transferred from one body to another, but the total store of mechanical energy remains unchanged until an external force does work.

Experiments and calculations show that if only elastic forces and forces of gravity act (in the absence of friction), the total potential and kinetic energies of a body or a system of bodies remain constant in all cases. This is the essence of the law of conservation of mechanical energy.

Using law of conservation of mechanical energy

Let me show how the law of mechanical energy conservation works with the help of the following example.

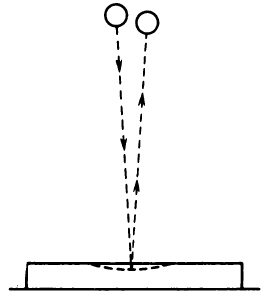
Consider a steel ball which falls onto a steel or glass slab strikes against it jumps up to the same height from which it fell. During the motion of the ball, a number of energy conversions take place. During its fall the potential energy of the ball is transformed into kinetic energy. When it touches the slab, both the ball and the slab start to deform. The kinetic energy is transformed into the potential energy of elastic deformation ( Like spring ) of the ball and the slab, this process is continued until the ball stops, i.e. until its entire kinetic energy is converted into potential energy of elastic deformation. Then under the action of elastic forces of the deformed slab, the ball acquires an upward velocity: the potential energy of elastic deformation of the slab and the ball has been converted into the kinetic energy of the ball. In the further upward motion, the velocity of the ball decreases under the action of the force of gravity, and the kinetic energy is converted into the potential energy of gravitation.

At the uppermost point, the ball again has only potential energy of gravitation. Since we can assume that the ball ascended to the same height from which it fell, the potential energy of the ball will be the same at the beginning and end of the process under consideration.

Moreover, the sum of the potential energy of gravitation, the potential energy of elastic deformation and kinetic energy remains the same at any instant of time and despite all energy transformations. The law of conservation of mechanical energy is fulfilled.