What is kinetic energy?

LEARNING OBJECTIVE 3.1.A - Describe the translational kinetic energy of an object in terms of the object’s mass and velocity.
AP MECHANICS -C LEARNING OBJECTIVE INT 4.C -. Calculate the change in kinetic energy due to the work done on an object or a system by a single force or multiple forces.

In the last section, we looked at ways to store work. The next obvious question is how to transport this work from one place to another.

Let’s consider the following experiment. Suppose we have a small box placed on a table. If we want to move this box, I can use my hand to move it. In the process we will apply some force on the box and also move it through some distance, hence, doing some work on the box. The question is how do we move the box without touching it or rather from a distance of 10 m or further?

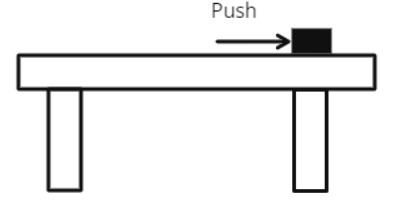
Alternatively, I can push a hard ball at some distance, doing work on it. This ball then rolls and pushes the box.

What has happened is something magical. I did some work on the ball and the ball seemed to store that work and further transported it to the box far away.

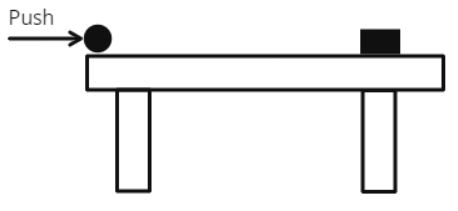
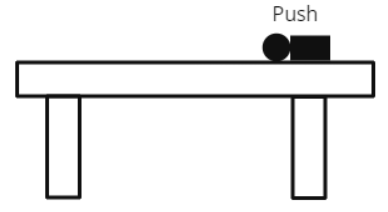
It appears the first place to store and transport the work is the motion itself.

Motion can store and transport work

Work involved motion (since an object had to be moved through a distance), so it is not surprising that motion could do work. It is moving air or wind, that drives a ship, and not still air; moving water that could turn a turbine, and not still water. It is not air or water that contained energy then, but the motion of the air or water. In fact, anything that moved contained energy, for if the moving object, whatever it collided with another, could transfer its energy to that second object and set its mass into motion; it would thus be doing work upon it.

English physicist Lord Kelvin in 1856, named the energy associated with motion as “kinetic energy”.

Kinetci energy

The word “kinetic” is from a Greek word meaning “motion.”

How much energy does a moving object contain?

Once it became clear that moving objects possess and transport energy the next question is how much energy a moving object contains while moving with a speed v?

To answer this question – let’s think in reverse order. The object imparted with a velocity does the work on other bodies coming in contact with it. So how much work in the first place is needed to impart a velocity v to the object? And exactly should be the Kinetic energy of the object, which will convert to work upon impact.

How to calculate kinetic energy ?

Let’s say that initially, the object of mass m is at rest on a frictionless floor. Let’s say we do a work amounting to W on the object, as a result, the object starts to move with a velocity V. Suppose the mass of the object is m, and the force we applied while doing work is F and the object moved a distance S before gaining the final velocity V.

Applying Newton’s law to the object we can write:

$F=ma$

Whereas Work will be:

$W = Fs = m \times ( as )$

Using the basic kinematical equation for motion in a straight line we know that $v^2= u^2 + 2as$, given the ball was initially at rest u = 0,

$v^2 = 2 as \mbox{ hence, } as = \frac{v^2}{2}$

substituting it in the previous equation will give:

$W = \frac{mv^2}{2}$

This is exactly the kinetic energy of the object of mass m moving with velocity v. So we have arrived at the expression to calculate the kinetic energy or in short KE:

$\mbox{Kinetic Energy} = KE = \frac{mv^2}{2}$

Important points about Kinetic Energy

• KE depends upon m and the square of velocity v.
• Both mass m and v are intrinsic properties of the object itself.
• Since mass m is a number and the $v^2$ is also a number, not a vector, given $v^2$ will always be positive or zero, KE of an object can never be a negative number. It can be zero if the v is zero or a positive number.
• KE is larger if the object’s mass is larger or its velocity is larger.
• Unit of KE will $kg(m/s)^2$ = Joules, same as work.
• Kinetic Energy is equivalent to Work.